Frequency of the Fluttering Crust
Method 1. We can approximate the fluttering frequency of the crust by modeling a narrow section of the crust as a frictionless granite piston of mass M and density rg compressing water of density rw . The piston, with an area A and thickness t, is free to vibrate up and down, much like a massive ship bobbing up and down on the sea.
The piston’s mass is
M = A t rg
A downward displacement of the crust by distance +x will produce an unbalanced restoring force (F), in the negative direction, of
F = - (rw g x) A
where g is the acceleration due to gravity. Therefore, using Newton’s second law, the equation of vertical motion is
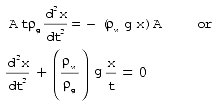
The solution to this differential equation can be written as
x = a sin w (t - t0)
where “a” is the amplitude, t is the time at any instant, t0 is the time when the crust is at its equilibrium position and moving downward, and the natural frequency w is
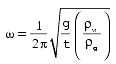
Therefore, the period (P) is
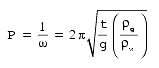
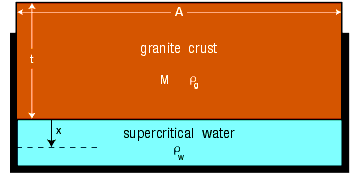
Figure 18: Vibrating Crust. While the fluttering crust would look like a flag held horizontally in a strong wind, its chaotic movement can be simplified by looking at the narrow section shown above. Its movement would primarily be up and down, driven by the oscillating pressure below.
From the steam table for supercritical water (SCW) mentioned in Endnote
14 on page
211, the density ( rw ) of SCW at 25,550 bars and 3,000°F is about![]() . If
. If
the vibrational period is 7.5 minutes per cycle. Other factors, such as water hammers, the partially removed pillars, and the stiffness of the crust could greatly alter this period.
Method 2. Analyses exist for the vibration of flat plates. [See for example Carl Roger Freberg and Emory N. Kemler, “Vibration of Thin Flat Plates,” Elements of Mechanical Vibration, 2nd edition (New York: John Wiley & Sons, Inc., 1949, pp. 147–148.] While these models have the advantage of incorporating a plate’s thickness and stiffness (modulus of elasticity), one must know the distance between supports, which for hydroplates requires knowing the number and spacing of the preflood pillars. While any estimate would be hard to justify, I will assume there were 18,000 evenly spaced pillars before the flood—the estimate previously used in Endnote 2 on page 474. With this spacing, these models give vibrational periods slightly greater than 43 minutes per cycle. Although uncertainties exist with both methods, the vibrational periods will be considered to be about 30 minutes.