Rapid Attraction
Two electrical charges (Q1 and Q2 statcoulombs, one positive and the other negative) are attracted toward each other by a force of F dynes when they are separated by a distance of x centimeters in a medium with permittivity k.
For a vacuum, k = 1. One statcoulomb is the charge of 2.08 × 109 electrons.
Stokes’ law gives the terminal velocity of a sphere of mass m and radius r which is acted upon by a force F in a fluid whose viscosity is m. That velocity is

The sphere’s density, r, is
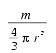
These equations simplify to
Integrating this from an initial separation distance of x0 until the charged particles collide (x = 0) at time t gives:

What does this mean? Consider trillions of radon-222 (222Rn) atoms flowing for weeks between sheets of mica that are growing, because the mineral-rich water’s temperature and pressure are dropping. If 222Rn (half-life = 3.8 days) ejects an alpha particle (charge = +2), the radon instantly becomes 218Po with a charge of Q1 = –2 and a radius r = 5 × 10-8 centimeters. That polonium ion will recoil with enough energy to remove hundreds of hydroxide ions (OH-) — each with a negative charge — from near the impact point in the mica. [For an explanation of dehydroxylation, see Endnote 130 on page 426.] While the water might absorb some recoil energy, or the polonium might be deflected off a mica sheet, some recoiling 218Po will crash into and become embedded in the mica, removing hundreds of hydroxide ions. This will give the impact point a large positive charge—both from the impact and the greater heating minutes later when the embedded 218Po decays by emitting an alpha particle.
Let’s conservatively say that the first impact in the mica produces a charge of Q 2 = +100. For water,
Other flowing 222Rn atoms that decay near that +100 point charge will be pulled into it within one 218Po half-life (3.1 minutes) if
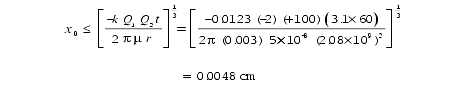
This is more than twice the radius of a 218Po halo. As more radon decays near the impact point and as more 218Po, 214Po, and 210Po are pulled into the impact point and then decay, the heating and recoil pressure remove more hydroxide ions, increasing the electrical charge Q 2. That, in turn, increases the distance, x0 and the rate at which polonium is pulled in. A runaway situation quickly develops.
The formula for biotite is K(Mg,Fe)3(Al,Fe)Si3O10(OH,F)2. Approximately 17/400 of its mass is OH- (highlighted in bold above). A typical inclusion at the center of a polonium halo has a radius of about 0.00005 cm. Therefore, that tiny volume of biotite, whose density is 3.1 gm/cm3, initially had about
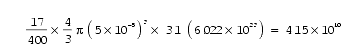
OH- ions.
If dehydroxylation removed only 1/20th of these ions, about a billion polonium ions could be attracted and concentrated, enough to form a sharp halo.
PREDICTION 57: A sensitive mass spectrometer will show about a 5% deficiency in hydrogen within the inclusions at the center of isolated polonium halos.